Answer:
The measure of
 is 30°.
is 30°.
Explanation:
In the statement, the angle has been misrepresented. The corrected statement is described below:
"On a unit circle, the terminal point of
 is
is
 . What is
. What is
 ?"
?"
The measure of the angle (
 ), in radians, is in standard form, that is, it is done with respect to the +x semiaxis. The measure of the angle whose terminal point is of the form
), in radians, is in standard form, that is, it is done with respect to the +x semiaxis. The measure of the angle whose terminal point is of the form
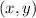 is determined by the following inverse trigonometric function:
is determined by the following inverse trigonometric function:
 (1)
(1)
If we know that
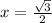 and
and
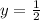 , then the measure of
, then the measure of
 is:
is:
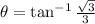
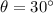
The measure of
 is 30°.
is 30°.