Answer:
Your given polynomial has only one real root which is x= -4
Explanation:
find any zero of the polynomial.
x-a divides the polynomial where a is a zero of polynomial:
So x+4 divides
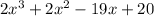
If we factor out a two, we can use the quadratic formula.
2(x^2-3x+2.5) so we have x = (-(-3)+/-(9-4*1*2.5)^(1/2))/2*1)=(3+i) or (3-i)/2 .
Final answer:
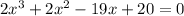
then x=-4, (3+i)/2, or (3-i)/2