
- Remember : To find the equation of straight line passing through given points ( x₁ , y₁ ) and ( x₂ , y₂ ) , Use
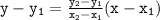
- Let the given points ( -7 , -1 ) be ( x₁ , y₁ ) & ( -7 , 12 ) be ( x₂ , y₂ ).

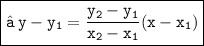
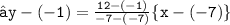
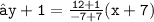

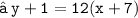




- Hence , 12x - y + 95 = 0 is the required equation of the line.
✺ STUDY HARD ! No matter if it seems impossible , no matter if it takes time , no matter if you have to wake up all night , Just remember that the feeling of success is the best thing in the entire world. ♪
۵Hope I helped ! ツ
☼Have a wonderful day / evening ! ☃
# StayInAndExplore ! ☂
▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁▁