Question

A $20, 000 brand new car depreciate its value every year following the pattern, $16,000, $12,800, $10,240. How much will the car be after 5 years?

A.$10, 000
B.$8,192
C.$6553.6
D.$6000

Answer 1

Explanation:

Use the info given in the exponential equation to find the value of b, the rate of decay.

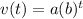`v(t)=a(b)^t` where v(t) is the value of the car after a certain number of years, t, have gone by, a is the initial value, and b is the rate of decay. We have everything we need but b:

a = 20000

v(t) = 16000 after t = 1 year:

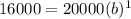`16000=20000(b)^1` so

b = .8 Taken in context, this means that the car depreciates 20% each year. Now we can solve the problem being asked of us, which is to find the value of the car after t = 5 years:

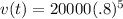`v(t)=20000(.8)^5` which simplifies down a bit to

v(t) = 20000(.32768) so

v(t) = 6553.60, choice C.