Question

One of the solutions to the equation x² - 5x-24 = 0 is 8. What is the other solution?​

Answer 1

-3

Explanation:

This is a quadratic equation. Therefore, one way we can solve this is by using the quadratic formula,
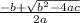`(-b+√(b^2-4ac) )/(2a)` and
`(-b-√(b^2-4ac) )/(2a)`.

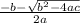a, b, and c represent the coefficients in our equation
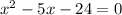`x^2-5x-24=0`. a represents the coefficient of the term with degree of 2, which is 1. b represents the coefficient of the term with degree of 1, which is -5. c represents the coefficient of the term with degree of 0, which is -24.

Let us plug them in the equation! Let us start with this one:
`(-b+√(b^2-4ac) )/(2a)`

`(5+√((-5)^2-4*1*(-24)) )/(2) =\\(5+√(25+96) )/(2) =\\(5+√(121) )/(2) =\\(5+11)/(2) =\\(16)/(2) =\\8`

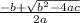
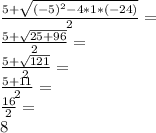
Oops! We are looking for the other solution. Let us plug in the values in this equation instead:
`(-b-√(b^2-4ac) )/(2a)`

`(5-√((-5)^2-4*1*(-24)) )/(2) =\\(5-√(25+96) )/(2) =\\(5-√(121) )/(2) =\\(5-11)/(2) =\\(-6)/(2) =\\-3`

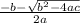
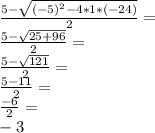
I hope this helps! Let me know if you have any questions :)

Answer 2

X^2 - 5x - 24
(X - 8)(x + 3)
X = 8 and x = -3
Therefore, the other solution is -3.