Given:
Consider the below figure attached with this question.
In circle A below, chord BC and diameter DAE intersect at F.
The arc CD = 46° and arc BE = 78°.
To find:
The measure of angle BFE.
Solution:
According to intersecting chords theorem, if two chords intersect inside the circle then the angle on the intersection is the average of intercepted arcs.
Using intersecting chords theorem, we get
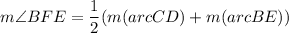
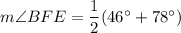
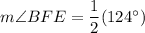
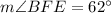
Therefore, the measure of angle BFE is 62°.