Given:
Area of a rectangle =
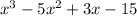
Width of rectangle =
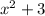
To find:
The length of the rectangle.
Solution:
The area of a rectangle is:
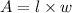
Where, l is length and w is the width.
It is can be written as:
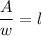

Putting
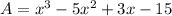 and
and
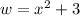 , we get
, we get
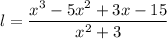

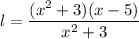

Therefore, the length of the rectangle is
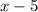 .
.