Answer:
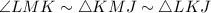
Explanation:
In the right triangle shown, two right triangles are inscribed in a third right triangle, with all three triangles sharing an altitude. Thus, all three right triangles are similar.
The second portion asks to draw them so that their corresponding sides and angles have the same orientation. I can't demonstrate that here but here are some tips:
- Draw them so that it is clear that they are similar
- Make sure that their corresponding sides match up
- When writing your similarity statement, the order of which you list the triangles' vertices should be maintained, e.g.
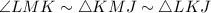
Here is an example of the drawing portion from the CK-12 Foundation: