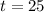Question

City A had a population of 10000 in the year 1990. City A’s population grows at a constant rate of 3% per year. City B has a population that is growing exponentially. In the year 2000, there were half as many people in B as in A. In the year 2010, the population of A was 20% more than the population of B.

When will the populations be equal? Give your answer in years after 1990.

Answer 1

City A and city B will have equal population 25years after 1990

Explanation:

Given

Let

`t \to` years after 1990

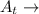`A_t \to` population function of city A

`B_t \to` population function of city B

City A

`A_0 = 10000` ---- initial population (1990)

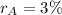`r_A =3\%` --- rate

City B

`B_(10) = (1)/(2) * A_(10)` ----- t = 10 in 2000

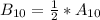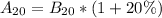`A_(20) = B_(20) * (1 + 20\%)` ---- t = 20 in 2010

Required

When they will have the same population

Both functions follow exponential function.

So, we have:

`A_t = A_0 * (1 + r_A)^t`

`B_t = B_0 * (1 + r_B)^t`

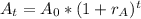
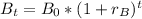
Calculate the population of city A in 2000 (t = 10)

`A_t = A_0 * (1 + r_A)^t`

`A_(10) = 10000 * (1 + 3\%)^(10)`

`A_(10) = 10000 * (1 + 0.03)^(10)`

`A_(10) = 10000 * (1.03)^(10)`

`A_(10) = 13439.16`

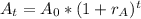
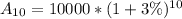
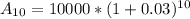
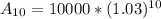
Calculate the population of city A in 2010 (t = 20)

`A_t = A_0 * (1 + r_A)^t`

`A_(20) = 10000 * (1 + 3\%)^(20)`

`A_(20) = 10000 * (1 + 0.03)^(20)`

`A_(20) = 10000 * (1.03)^(20)`

`A_(20) = 18061.11`

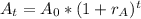
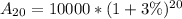
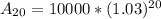
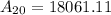
From the question, we have:

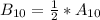`B_(10) = (1)/(2) * A_(10)` and
`A_(20) = B_(20) * (1 + 20\%)`

`B_(10) = (1)/(2) * A_(10)`

`B_(10) = (1)/(2) * 13439.16`

`B_(10) = 6719.58`

`A_(20) = B_(20) * (1 + 20\%)`

`18061.11 = B_(20) * (1 + 20\%)`

`18061.11 = B_(20) * (1 + 0.20)`

`18061.11 = B_(20) * (1.20)`

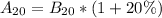
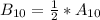
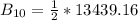
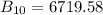
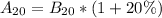
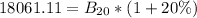
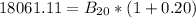
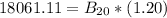
Solve for B20

`B_(20) = (18061.11)/(1.20)`

`B_(20) = 15050.93`

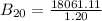
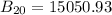
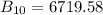`B_(10) = 6719.58` and
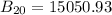`B_(20) = 15050.93` can be used to determine the function of city B

`B_t = B_0 * (1 + r_B)^t`

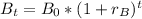
For:
`B_(10) = 6719.58`

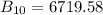
We have:

`B_(10) = B_0 * (1 + r_B)^(10)`

`B_0 * (1 + r_B)^(10) = 6719.58`

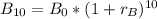
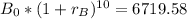
For:
`B_(20) = 15050.93`

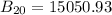
We have:

`B_(20) = B_0 * (1 + r_B)^(20)`

`B_0 * (1 + r_B)^(20) = 15050.93`

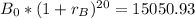
Divide
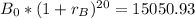`B_0 * (1 + r_B)^(20) = 15050.93` by
`B_0 * (1 + r_B)^(10) = 6719.58`

`(B_0 * (1 + r_B)^(20))/(B_0 * (1 + r_B)^(10)) = (15050.93)/(6719.58)`

`((1 + r_B)^(20))/((1 + r_B)^(10)) = 2.2399`

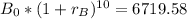
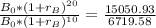
Apply law of indices

`(1 + r_B)^(20-10) = 2.2399`

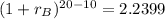
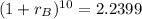`(1 + r_B)^(10) = 2.2399` --- (1)

Take 10th root of both sides

`1 + r_B = \sqrt[10]{2.2399}`

`1 + r_B = 1.08`

Subtract 1 from both sides

`r_B = 0.08`

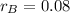
To calculate
`B_0`, we have:

`B_0 * (1 + r_B)^(10) = 6719.58`

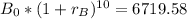
Recall that:
`(1 + r_B)^(10) = 2.2399`

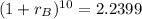
So:

`B_0 * 2.2399 = 6719.58`

`B_0 = (6719.58)/(2.2399)`

`B_0 = 3000`

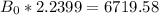
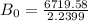
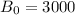
Hence:

`B_t = B_0 * (1 + r_B)^t`

`B_t = 3000 * (1 + 0.08)^t`

`B_t = 3000 * (1.08)^t`

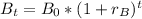
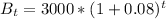
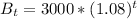
The question requires that we solve for t when:

`A_t = B_t`

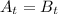
Where:

`A_t = A_0 * (1 + r_A)^t`

`A_t = 10000 * (1 + 3\%)^t`

`A_t = 10000 * (1 + 0.03)^t`

`A_t = 10000 * (1.03)^t`

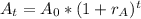
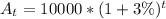
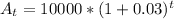
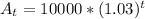
and

`B_t = 3000 * (1.08)^t`

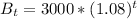
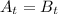`A_t = B_t` becomes

`10000 * (1.03)^t = 3000 * (1.08)^t`

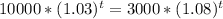
Divide both sides by 10000

`(1.03)^t = 0.3 * (1.08)^t`

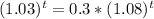
Divide both sides by
`(1.08)^t`

`((1.03)/(1.08))^t = 0.3`

`(0.9537)^t = 0.3`

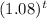
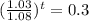
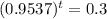
Take natural logarithm of both sides

`\ln(0.9537)^t = \ln(0.3)`

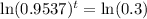
Rewrite as:

`t\cdot\ln(0.9537) = \ln(0.3)`

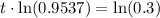
Solve for t

`t = (\ln(0.3))/(ln(0.9537))`

`t = 25.397`

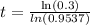
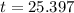
Approximate

`t = 25`