Answer: The frequency of the green light emitted by a hydrogen atom with a wavelength of 546 nm is
 .
.
Step-by-step explanation:
Given: Wavelength = 546 nm
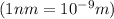 =
=
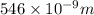
The relation between frequency and wavelength is as follows.
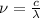
where,
 = frequency
= frequency
c = speed of light =
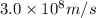
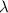 = wavelength
= wavelength
Substitute the values into above formula as follows.
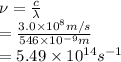
Thus, we can conclude that the frequency of the green light emitted by a hydrogen atom with a wavelength of 546 nm is
 .
.