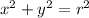Question

Find an equation of the circle that satisfies the given conditions. (Use the variables x and y.)

Center at the origin;
passes through (4, 6)

Answer 1

`x^2 + y^2 = 52`

Explanation:

Distance between two points:

Suppose that we have two points,
`(x_1,y_1)` and
`(x_2,y_2)`. The distance between them is given by:

`D = √((x_2-x_1)^2+(y_2-y_1)^2)`

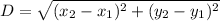
Equation of a circle:

The equation of a circle with center
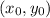`(x_0,y_0)` and radius r has the following format:

`(x - x_0)^2 + (y - y_0)^2 = r^2`

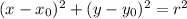
Center at the origin;

This means that
`x_0 = 0, y_0 = 0`

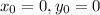
So

`(x - x_0)^2 + (y - y_0)^2 = r^2`

`(x - 0)^2 + (y - 0)^2 = r^2`

`x^2 + y^2 = r^2`

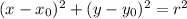
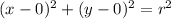
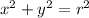
Passes through (4, 6)

The radius is the distance from this point to the center. So

`r = √((x_2-x_1)^2+(y_2-y_1)^2)`

`r = √((4-0)^2+(6-0)^2)`

`r = √(16+36)`

`r = √(52)`

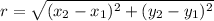
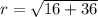
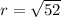
So

`r^2 = 52`

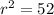
Then

`x^2 + y^2 = r^2`

`x^2 + y^2 = 52`