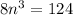Question

Let n be an odd integer with exactly 11 positive divisors. Find the number of positive divisors of 8n^3

Answer 1

Answer: 124

Explanation:

As we know, the total number of positive divisors of n is the product of one more than each exponent in the prime factorization of n.

We know this product is equal to 11.

Since 11 is prime, so there can be only one number in this product, so the prime factorization of n must be
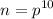`$n=p^(10)$`, where p is a prime number.

We take a cube of the prime factorization of n to give us a prime factorization for
`n^3`

`$$n^(3)=\left(p^(10)\right)^(3)=p^(30) .$$`

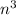
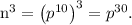
We see that
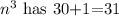`n^(3)\text{ has }$30+1=31$` positive divisors.

Now,
`8n^3=2^3* p^(30)`

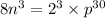
Totald divisiors =
`(3+1)(30+1)=4*31=124`

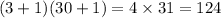
Hence, the number of positive divisors of
`8n^3=124`