Question

A 5 kg box drops a distance of 10 m to the ground. If 70% of the initial potential energy goes into increasing the internal energy of the box, determine the magnitude of the increase.

Answer 1

Step-by-step explanation:

From the given information:

The initial PE
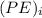`(PE)_i` = m×g×h

= 5 kg × 9.81 m/s² × 10 m

= 490.5 J

The change in Potential energy P.E of the box is:

ΔP.E =
`P.E_f -P.E_i`

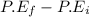
ΔP.E = 0 -
`(PE)_i`

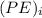
ΔP.E =
`-P.E_i`

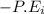
If we take a look at conservation of total energy for determining the change in the internal energy of the box;

`\Delta P.E + \Delta K.E + \Delta U = 0`

`\Delta U = -\Delta P.E - \Delta K.E`

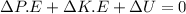
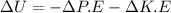
this can be re-written as:

`\Delta U =- (-\Delta P.E_i) - \Delta K.E`

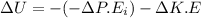
Here, K.E = 0

Also, 70% goes into raising the internal energy for the box;

Thus,

`\Delta U =(70\%) \Delta P.E_i-0`

`\Delta U =(0.70) (490.5)`

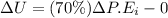
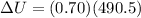
ΔU = 343.35 J

Thus, the magnitude of the increase is = 343.35 J