Question

Hypochlorous acid decays in the presence of ultraviolet radiation. Assume that degradation occurs accord- ing to first-order kinetics and the rate of degradation was measured to be 0.12 day−1 (at a particular sun- light intensity and temperature). Given this, how long does it take for the concentration of hypochlorous acid to reach nondetectable levels (0.05 mg · L−1) if the initial concentration were 3.65 mg · L−1?

Answer 1

35.75 days

Step-by-step explanation:

From the given information:

For first-order kinetics, the rate law can be expressed as:

`\mathsf{In (C)/(C_o) = -kt}`

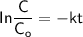
Given that:

the rate degradation constant = 0.12 / day

current concentration C = 0.05 mg/L

initial concentration C₀ = 3.65 mg/L

`\mathsf{In( (0.05)/(3.65))= -(0.12) t}`

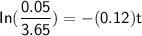
㏑(0.01369863014) = -(0.12) t

-4.29 = -(0.12)

t = -4.29/-0.12

t = 35.75 days