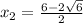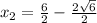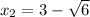Answer:
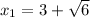
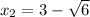
Explanation:
Given the quadratic equation;
x² - 6x + 3 = 0
To find the roots of the quadratic equation, we would use the quadratic formula;
Note: the standard form of a quadratic equation is ax² + bx + c = 0
a = 1, b = -6 and c = 3
The quadratic equation formula is;

Substituting into the formula, we have;
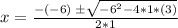
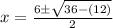

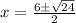
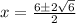
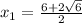
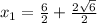
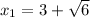
Or