Answer:
Explanation:
Because angle NRM is vertical to angle QRP, they are congruent. That means that arc NM is congruent to arc PQ, making arc MQ and arc NP congruent. That means that arc NP is also 162. Since the outside of a circle measures 360 regardless of how big or small the circle is, we can find the measures of arcs MN and PQ by doing some simple math.
Arc MN = Arc PQ = 360 - 162 - 162 = 36 and divide that in half to get
Arc MN = Arc PQ = 18°. As we all know, the measure of a central angle is congruent to the measure of the arc it intercepts, so the measure of angle NRM is 18°. That gives us the angle we need for the formula:
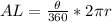 where θ is the measure of the central angle, 18°:
where θ is the measure of the central angle, 18°:
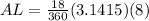 which gives us
which gives us
AL = 1.26 feet (Note: arc length is in distance measures, like feet, inches, meters, etc., while arc measure will always be in degrees!)