Final Answer:
1. The order of transformation for the graph of the function
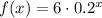 compared to the base function
compared to the base function
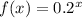 is a vertical stretch by a factor of 6.
is a vertical stretch by a factor of 6.
Step-by-step explanation:
The given function is
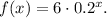 To understand the transformation, let's compare it with the base function
To understand the transformation, let's compare it with the base function
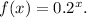 The general form for a vertical stretch or compression is
The general form for a vertical stretch or compression is
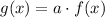 , where
, where
 is the stretch or compression factor.
is the stretch or compression factor.
In this case,
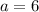 , which means there is a vertical stretch by a factor of 6. The calculation involves evaluating the function for specific values of
, which means there is a vertical stretch by a factor of 6. The calculation involves evaluating the function for specific values of
 and observing the corresponding
and observing the corresponding
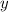 values. For example, if
values. For example, if
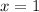 , in the base function,
, in the base function,
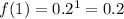 , and in the transformed function,
, and in the transformed function,
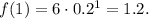 This multiplication by 6 represents the vertical stretch.
This multiplication by 6 represents the vertical stretch.
The order of transformation is determined by the sequence in which multiple transformations occur. In this case, there is a single transformation—vertical stretching. The type of transformation is identified as a vertical stretch because the
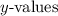 are stretched by a factor of 6, making the graph taller compared to the base function. Therefore, the final answer is a vertical stretch by a factor of 6.
are stretched by a factor of 6, making the graph taller compared to the base function. Therefore, the final answer is a vertical stretch by a factor of 6.