Answer:
65 Hz, 95 Hz, 150 Hz, 180 Hz, 310 Hz, 340 Hz
Step-by-step explanation:
Given :
Frequencies of the sinusoids,
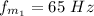 , and
, and
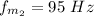
Sampling rate
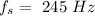
The positive frequencies at the output of the sampling system are :

When n = 0,

when n = 1,


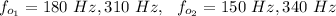
When n = 2,
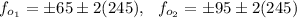
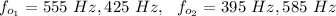
Therefore, the first six positive frequencies present in the replicated spectrum are :
65 Hz, 95 Hz, 150 Hz, 180 Hz, 310 Hz, 340 Hz