Note: Consider we need to find the amount.
Given:
Principal = $38000
Rate of interest = 9% compounded monthly
Time = 7 years
To find:
The amount after 7 years.
Solution:
Formula for amount:
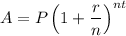
Where, P is principal, r is the rate of interest in decimal, n is the number of times interest compounded in an year and t is the number of years.
The interest is compounded monthly, so n=12.
Putting
 in the above formula, we get
in the above formula, we get
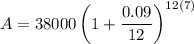

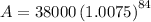
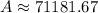
Therefore, the amount after 7 years is $71181.67.