Answer:
The level in decibels is 100.
Explanation:
The intensity level in decibels (β) can be found using the following equation:
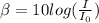
Where:
I: is the intensity = 10⁻⁶ W/cm²
I₀: is the reference intensity = 10⁻¹² W/m²
First, we need to convert the unit of the given intensity from W/cm² to W/m².
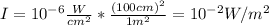
Hence, the level in decibels is:

Therefore, the level in decibels is 100.
I hope it helps you!