Answer:

Explanation:
Parallelogram is a quadrilateral whose both pairs of opposite sides are parallel and equal and opposite angles are also equal.

So,

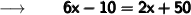
Subtracting 2x from both sides we get :
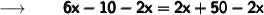
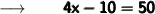
Adding 10 to both sides we get :
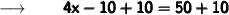
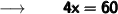
Dividing 4 from both sides we get :
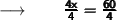

Therefore,