Answer:
Explanation:
There are several things we need to do/know to figure this out. First we have to know that the perpendicular slope to a line is the opposite reciprocal of the slope of which it is perpendicular (did that make sense?!). In other words, we have to find the slope of the line 5x - 8y + 24 = 0 and then take the opposite reciprocal of it. Solving for y:
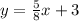 with a slope of 5/8. The perpendicular slope, then, is -8/5. So we have m now in y = mx + b. Now onto the second equation...
with a slope of 5/8. The perpendicular slope, then, is -8/5. So we have m now in y = mx + b. Now onto the second equation...
The equation for which we need the same x-intercept is 7x - 12y - 70 = 0. The x-intercept exists when y = 0, so filling in a 0 for y:
7x - 12(0) = 70 and
x = 10. Now we know x. We also know, however, that when x = 10, y = 0, so we have everything we need to sub into the linear equation and solve for b:
0 = -8/5(10) + b and
0 = -16 + b so
b = 16 and the equation we are looking for is
y = -8/5x + 16