Question

A mixture containing nitrogen, hydrogen, and iodine established the following equilibrium at 400 °C:2NH3(g) + 3I2(g) ⇌ N2(g) + 6HI(g)Use the information below to calculate Kc for this reaction.N2(g)+3H2(g)⇌2NH3(g) Kc1= 0.50 at 400CH2(g)+I2(g)⇌2HI(g Kc2= 50 at 400°C

Answer 1

Answer: The value of
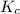`K_(c)` for this reaction is 250000.

Step-by-step explanation:

The given equation is as follows.

`2NH_(3)(g) + 3I_(2)(g) \rightleftharpoons N_(2)(g) + 6HI(g)`

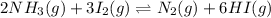
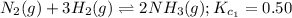`N_(2)(g) + 3H_(2)(g) \rightleftharpoons 2NH_(3)(g); K_{c_(1)} = 0.50` ... (1)

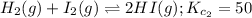`H_(2)(g) + I_(2)(g) \rightleftharpoons 2HI(g); K_{c_(2)} = 50` ... (2)

To balance the atoms, multiply equation (2) by 3. Hence, the equation (2) can be re-written as follows.

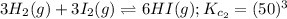`3H_(2)(g) + 3I_(2)(g) \rightleftharpoons 6HI(g); K_{c_(2)} = (50)^(3)` ... (3)

Now, subtract equation (1) from equation (3). So, the equation formed will be as follows.

`3I_(2) - N_(2) \rightleftharpoons 6HI - 2NH_(3)`

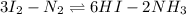
This equation can also be re-written as follows.

`3I_(2) + 2NH_(3) \rightleftharpoons N_(2) + 6HI`

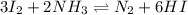
This equation is similar to the equilibrium equation given to us.

Therefore, during this subtraction the equation constants get divided as follows.

`K^(')_(c) = \frac{K_{c_(2)}}{K_{c_(1)}}\\= ((50)^(3))/(0.50)\\= 250000`

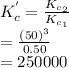
Thus, we can conclude that the value of
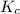`K_(c)` for this reaction is 250000.