Question

For the sample standard deviation(s) for situations involving means, explain whether it affects the width of a confidence interval, the center of a confidence interval, neither, or both.

Answer 1

Affects the width of a confidence interval, as the margin of error is a function of the sample standard deviation.

Explanation:

When we have the standard deviation for the sample, the t-distribution is used to solve the question.

It does not affect the center of the interval, which is a function only of the sample mean.

Width of a confidence interval:

The width of a confidence interval is a function of the margin of error, which is:

`M = t(s)/(√(n))`

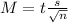
In which s is related to the sample standard deviation. Thus, since s and M are directly proportional, a higher standard deviation makes the interval wider, lower standard deviation makes the interval narrower, and thus, the sample standard deviation affects the the width of a confidence interval.