Question

A farmer's silo is in the shape of a cylinder topped by a hemisphere. If the radius of the silo is 13 ft and the height of the cylindrical portion is 44 ft, what is the volume of the silo?

Answer 1

V = 27948.09 cubic feet

Explanation:

Given that,

A farmer's silo is in the shape of a cylinder topped by a hemisphere.

The radius of silo, r = 13 ft

Height of the cylindrical portion, h = 44 ft

We need to find the volume of the silo. Net volume is equal to :

V = Volume of cylinder + volume of hemisphere

i.e.

`V=\pi r^2h+(2)/(3)\pi r^3`

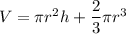
Put all the values,

`V=3.14* 13^2* 44+(2)/(3)* 3.14* 13^3\\\\V=27948.09\ ft^3`

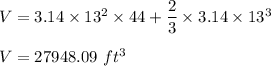
Hence, the volume of the silo is equal to 27948.09 cubic feet.