Question

Pls help asappppppp it is very important I need it pls

Answer 1

Given:

The graph of a proportional relationship.

To find:

The constant of proportionality, the value of y when x is 24 and the value of x when y is 108.

Solution:

If y is directly proportional to x, then

`y\propto x`

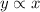
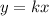`y=kx` ...(i)

Where, k is the constant of proportionality.

The graph of proportional relationship passes through the point (5,15).

Substituting x=5 and y=15 in (i), we get

`15=k(5)`

`(15)/(5)=k`

`3=k`

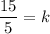
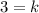
Therefore, the constant of proportionality is 3.

Substituting k=3 in (i) to get the equation of the proportional relationship.

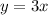`y=3x` ...(ii)

Substituting x=24 in (ii), we get

`y=3(24)`

`y=72`

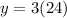
Therefore, the value of y is 72 when x is 24.

Substituting y=108 in (ii), we get

`108=3x`

`(108)/(3)=y`

`36=y`

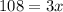
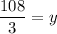
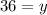
Therefore, the value of x is 36 when y is 108.