Final answer:
The total amount in Noah's account after two years is approximately $3672.35.
Step-by-step explanation:
To find the total amount in Noah's account after two years, we can use the formula for compound interest:
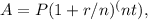
where A is the future amount, P is the principal (initial investment), r is the annual interest rate (0.43% in this case), n is the number of times interest is compounded per year (12 in this case), and t is the number of years (2 in this case).
Substituting the given values, we get:
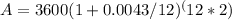
Solving this equation, the total amount in Noah's account after two years is approximately $3672.35.