Question

A non-conducting spherical thick shell, of inner radius a and outer radius 2a, carries a charge per unit volume density. Calculate the electrostatic potential energy of this system g

Answer 1

Answer: Electrostatic potential energy of the given system is

U=1/4π∈*(96/5)*Q²/a

Step-by-step explanation:

consider spherical shell of inner radius a (taken as x) and outer radius 2a ,carrying charge Q and volume charge density ρ=Q/4π(2a)³...........................(1)

then voume of the shell=4πx²dx

charge on the shell inner part of the shell.q1=4πx²dx ρ

charge on this ,in case of sphereical type,q2=4/3*πx³ ρ

then electrostatic energy of sperical charge of radius a (which we taken as x) and sperical shell of radius x and thickness dx is,

dU=1/4π∈ *(q1q2)/x

by placing value of q1 and q2 from above , we will get a equation

dU=1/4π∈*(16/3*π²ρ²x
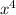`x^(4)`dx

then total electrostatic energy of sperical charge by integrating from limit a to 2a and putting value of ρ then we will get

U=1/4π∈*(96/5)Q²/a