Question

Instead of changing the the frequency you can change the tension to produce the next higher harmonic. What percentage of the tension would produce the next higher harmonic?

Answer 1

`(T)/(T_o) = ( 1 + (1)/(n) )^2`

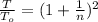
Step-by-step explanation:

This is a string resonance exercise, the wavelengths in a string held at the ends is

λ = 2L₀ / n

where n is an integer

the speed of the wave is

v = λ f

f = v /λ

the speed of the wave is given by the characteristics of the medium (string)

v =
`\sqrt{(T)/(\mu ) }`

we substitute

f =
`(n)/(2L_o) \ \sqrt{(T)/(\mu ) }`

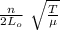
to obtain the following harmonic we change n → n + 1

f’ =
`(n+1)/(2L_o) \ \sqrt{(T_o)/(\mu ) }`

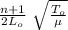
In this case, it tells us to change the tension to obtain the same frequency.

f ’= \frac{n}{2L_o} \ \sqrt{\frac{T}{\mu } }

how the two frequencies are equal

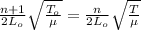
`(n+1)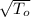/(2L_o) \sqrt{(T_o)/( \mu ) } = (n)/(2L_o) \sqrt{(T)/(\mu ) }`

(n + 1)
`√(T_o)` = n
`√(T)`

`(T)/(T_o) = ( (n+1)/(n) )^2`

`(T)/(T_o) = ( 1 + (1)/(n) )^2`

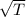
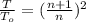
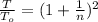
this is the relationship of the voltages to obtain the following harmonic,