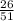Answer:
(a)
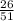
(b)
Explanation:
(i) Probability is the likelihood of whether or not an event will occur. It is given by the ratio of the number of favourable outcomes to the number of expected outcomes. i.e
P = number of favourable outcomes / total number of possible outcomes
For drawing a first card which is black,
number of favourable outcomes = 26 (since there are a total of 26 black cards in a deck of card)
total number of possible outcomes = 52 (since the total number of cards in a deck of card is 52)
∴ Probability of drawing the first black card = 26 / 52 = 1 / 13
Since the second card is not a black card, it is a red card.
number of favourable outcomes = 26 (since there are a total of 26 red cards in a deck of card)
total number of possible outcomes = 51 (since a black card has been previously picked from the deck)
∴ Probability of drawing a second black card = 26 / 51
The probability that the second is not a black card is 26 / 51
(ii) The conditional probability of a given event B is the probability that the event will occur knowing that a previous event A has already occurred,
It is given by;
P(B|A) = P(A and B) ÷ P(A)
In this case;
event B is drawing a second card which is not black
event A is drawing a first card which is black
This implies that;
P(A and B) =
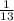 x
x
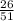
P(A) =
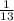
Substitute these values in the equation for conditional probability as follows;
P(B|A) =
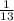 x
x
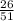 ÷
÷
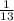
P(B|A) =
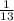 x
x
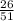 x
x
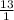
P(B|A) =
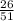
Therefore the conditional probability is