Answer:
0.2%
Explanation:
The given parameters are;
The percentage of product within the correct range = 95%
The percentage of the times the sensor reject boxes of incorrect weight = 98%
The percentage of the times the company reject boxes with correct weight = 1%
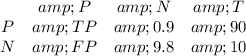
We have, FN = 0.9, TN = 9.8, TP = 90 - 0.9 = 89.1, FP = 10 - 9.8 = 0.2
FNR = FN/(FN + TP) = 0.9/(0.9 + 89.1) = 0.01
FPR = 0.2/(0.2 + 9.8) = 0.02
TPR = 89.1/(89.1 + 0.9) = 0.99
TNR = 9.8/(9.8 + 0.2) = 0.98
P(C/A) = TPR × P(C)/((TPR × P(C) + FPR×(1 - P(C)))
Where;
P(C/A) = The probability that a correct weight package is accepted
∴ P(C/A) = 0.99*0.9/(0.99*0.9 + 0.02*0.1) ≈ 0.99776
The probability that a correctly weighed package is rejected, P(C/R), is given as follows;
P(C/R) = 1 - P(C/A)
∴ P(C/R) ≈ 1 - 0.99776 = 0.00224 ≈ 0.2%
The probability that a correctly weighed package is rejected, P(C/R) ≈ 0.2%