Question

Mary is planning to survey a sample of women to find out how much money the average woman spent on lipstick last year. What sample size will she need, if she wants to be 95% confident that her sample mean is no more than $4 away from the population mean, and assuming a standard deviation of $20? A. 100

Answer 1

Answer: The required sample size = 97

Explanation:

If prior population standard deviation is known, then the minimum sample size can be computed as:

`n=((z^**\sigma)/(E))^2`

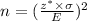
,where z* = critical z-value

`\sigma` = population standard deviation

E = Margin of error

As per given,

`\sigma=20,\ E= 4`

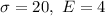
Critical value for 95% confidence = 1.96

`n=((1.96*20)/(4))^2\\= (1.96* 5)^2\\= (9.8)^2\\=96.04\approx97`

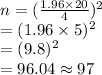
Hence, the required sample size = 97