Answer:
The p-value of the test is 0.209 > 0.05, which means that there is not enough evidence at the 0.05 level to support the manager's claim.
Explanation:
The company's promotional literature states that 44% of the chips fail in the first 1000 hours of their use. The quality control manager wants to test the claim that the actual percentage that fail is below the stated percentage.
At the null hypothesis, we test if the proportion is of 44%, the stated value. So
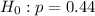
At the alternate hypothesis, we test if the proportion is below the stated value, that is, it is less than 0.44. So
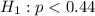
The test statistic is:

In which X is the sample mean,
 is the value tested at the null hypothesis,
is the value tested at the null hypothesis,
 is the standard deviation and n is the size of the sample.
is the standard deviation and n is the size of the sample.
0.44 is tested at the null hypothesis:
This means that
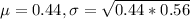
A sample of 1600 computer chips revealed that 43% of the chips fail in the first 1000 hours of their use.
This means that
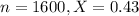
Value of the test statistic:

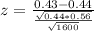
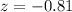
P-value of the test:
The p-value of the test is the probability of finding a sample proportion below 0.43, which is the p-value of z = -0.81.
Looking at the z-table, z = -0.81 has a p-value of 0.209.
The p-value of the test is 0.209 > 0.05, which means that there is not enough evidence at the 0.05 level to support the manager's claim.