This question is incomplete, the complete question is;
An air-standard Diesel cycle engine operates as follows: The temperatures at the beginning and end of the compression stroke are 30 °C and 700 °C, respectively. The net work per cycle is 590.1 kJ/kg, and the heat transfer input per cycle is 925 kJ/kg. Determine the a) compression ratio, b) maximum temperature of the cycle, and c) the cutoff ratio, v3/v2.
Use the cold air standard assumptions.
Answer:
a) The compression ratio is 18.48
b) The maximum temperature of the cycle is 1893.4 K
c) The cutoff ratio, v₃/v₂ is 1.946
Step-by-step explanation:
Given the data in the question;
Temperature at the start of a compression T₁ = 30°C = (30 + 273) = 303 K
Temperature at the end of a compression T₂ = 700°C = (700 + 273) = 973 K
Net work per cycle
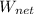 = 590.1 kJ/kg
= 590.1 kJ/kg
Heat transfer input per cycle Qs = 925 kJ/kg
a) compression ratio;
As illustrated in the diagram below, 1 - 2 is adiabatic compression;
so,
Tγ
 = constant { For Air, γ = 1.4 }
= constant { For Air, γ = 1.4 }
hence;
⇒ V₁ / V₂ =
 T₂ / T₁
T₂ / T₁
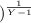
so we substitute
⇒ V₁ / V₂ =
 973 K / 303 K
973 K / 303 K
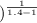
=
 3.21122
3.21122

= 18.4788 ≈ 18.48
Therefore, The compression ratio is 18.48
b) maximum temperature of the cycle
We know that for Air, Cp = 1.005 kJ/kgK
Now,
Heat transfer input per cycle Qs = Cp( T₃ - T₂ )
we substitute
925 = 1.005( T₃ - 700 )
( T₃ - 700 ) = 925 / 1.005
( T₃ - 700 ) = 920.398
T₃ = 920.398 + 700
T₃ = 1620.398 °C
T₃ = ( 1620.398 + 273 ) K
T₃ = 1893.396 K ≈ 1893.4 K
Therefore, The maximum temperature of the cycle is 1893.4 K
c) the cutoff ratio, v₃/v₂;
Since pressure is constant, V ∝ T
So,
cutoff ratio S = v₃ / v₂ = T₃ / T₂
we substitute
cutoff ratio S = 1893.396 K / 973 K
cutoff ratio S = 1.9459 ≈ 1.946
Therefore, the cutoff ratio, v₃/v₂ is 1.946