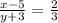Question

Two numbers are in the ratio 5:6. If the smaller one is decreased by 5 and

the other is increased by 3, the new ratio is 2:3. Find the number.​

Answer 1

Smallest Number = 35

Other Number = 42

Explanation:

Let the smaller number be x.

Let the other number be y.

Given:

x : y = 5 : 6

That is,

`(x)/(y) = (5)/(6)`

`=> 5y = 6x\\\\=>x = (5y)/(6)`

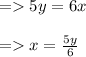
Next: Let the smaller number be reduced by 5, x - 5

Let the other number be increased by 3, y + 3

Given : (x - 5) : (y + 3) = 2 : 3

That is,

`(x-5)/(y+3) = (2)/(3)\\`

`(x-5) * 3 = (y+3)* 2\\\\substitute \ x = (5y)/(6)\\\\3((5y)/(6) -5) = 2y + 6\\\\(5y)/(2) - 15 = 2y + 6\\\\(5y)/(2) - 2y = 6 + 15\\\\(5y-4y)/(2) = 21\\\\y = 21 * 2 = 42\\\\Substitute \ y \ in \ x = (5y)/(6) \\\\x = (5 * 42)/(6) = 35`