Question

(10 points) help plz

Answer 1

(c) No; There is an exponent on a variable x that is not a whole number

Explanation:

Given

`f(x) = x^2 - \sqrt[5]{x}`

Required

Determine if the function is a polynomial function of not

A polynomial function is represented as:

`f(x) =ax^n + bx^(n-1) + cx^(n-2)+........+z`

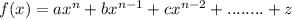
Where n is an integer and the least power is 0

So, we have:

`f(x) = x^2 - \sqrt[5]{x}`

The degree of x is
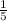`(1)/(5)` ----- i.e. not an integer

Hence, the function is not a polynomial