Question

Suppose you want to start saving for retirement. You decide to continuously invest $5000 of your income each year in a risk-free investment with a 7% yearly interest rate compounded continuously. If y| is the value of the investment, and t| is in years. dy/dt =| Your answer should be interms of y|. You start investing at t = 0| so y(0) = 0. y(t) =| What is the size of your investment after 40 years. y(40) =|

Answer 1

The size of the investment after 40 years is of $74,872.

Explanation:

Continuous compounding:

The amount of money, in continuous compounding, after t years, is given by:

`y(t) = y(0)(1+r)^t`

In which y(0) is the initial investment and r is the interest rate, as a decimal.

You decide to continuously invest $5000 of your income each year in a risk-free investment with a 7% yearly interest rate compounded continuously.

This means that
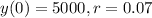`y(0) = 5000, r = 0.07`. So

`y(t) = y(0)(1+r)^t`

`y(t) = 5000(1+0.07)^t`

`y(t) = 5000(1.07)^t`

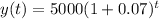
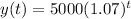
What is the size of your investment after 40 years?

This is y(40), that is, y when t = 40. So

`y(t) = 5000(1.07)^t`

`y(40) = 5000(1.07)^(40)`

`y(40) = 74872`

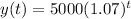
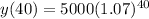
The size of the investment after 40 years is of $74,872.