Question

Weather balloons are filled with hydrogen and released at various sites to measure and transmit data about conditions such as air pressure and temperature. A weather balloon is filled with hydrogen at the rate of 0.5 ft^3/s. Initially, the balloon has 4 ft^3 of hydrogen. Initially, the balloon has 2 ft^3 of hydrogen.

Required:
a. Find a linear function V that models the volume of hydrogen in the balloon at any time t.
b. If the balloon has a capacity of 15 ft^3, how long does it take to completely fill the balloon?

Answer 1

a) The linear function that models the volume of hydrogen in the balloon at any time
`t` is
`V(t) = 2 + 0.5\cdot t`.

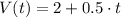b) 26 seconds are needed to completely fill the balloon.

Explanation:

The statement has a mistake, the correct form is described below:

Weather balloons are filled with hydrogen and released at various sites to measure and transmit data about conditions such as air pressure and temperature. A weather balloon is filled with hydrogen at the rate of
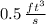`0.5\,(ft^(3))/(s)`. Initially, the balloon has
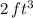`2\,ft^(3)` of hydrogen.

a) The volume of weather balloons is increasing linearly in time (
`t`), in seconds, since the rate of change of volume (
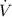`\dot V`), in cubic feet per second, is stable. The linear function of the volume of the weather balloon in terms of time is:

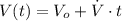`V(t) = V_(o) + \dot V\cdot t` (1)

Where:

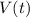`V(t)` - Current volume, in cubic feet.

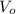`V_(o)` - Initial volume, in cubic feet.

If we know that
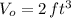`V_(o) = 2\,ft^(3)` and
`\dot V = 0.5\,(ft^(3))/(s)`, then the volume as a function of time is:

`V(t) = 2 + 0.5\cdot t`

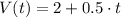
b) If we know that
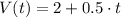`V(t) = 2 + 0.5\cdot t` and
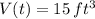`V(t) = 15\,ft^(3)`, then the time taken to fill the balloon is:

`V(t) = 2 + 0.5\cdot t`

`V(t) - 2 = 0.5\cdot t`

`t = (V(t) - 2)/(0.5)`

`t = (15-2)/(0.5)`

`t = 26\,s`

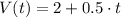
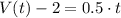
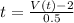
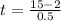
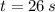
26 seconds are needed to completely fill the balloon.