Answer:
4.2 units
most likely answer choice B
Explanation:
(1, 2) and (4, 5)
To find the distance between two points, we use the distance formula:
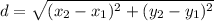
Let's plug in what we know.
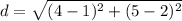
Evaluate the parentheses.
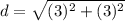
Evaluate the exponents.
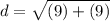
Add.
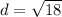
Evaluate the radical.
d = 4.24
Round to the nearest tenth.
d = 4.2 units
*note: The answer choice is 4.6. I'm not sure if that is a typo on someone's end, but the distance between these two points is exactly 4.24264068712 units.
Hope this helps!