Question

Please help ASAP IM IN DESPREATE NEED OF HELP

EVEN IF YOU COULD HELP WITH ONE I'D BE HAPPY I JUST DON'T UNDERSTAND WHAT THEY ARE ASKING.

Answer 1

See the attached image for the graph of the first system.

Explanation:

Here's how to graph the first system.

Start with the inequality
`-y \le -2x-3`. You can make this easier to work with by multiplying through by -1. Remember to switch the inequality sign when multiplying by a negative number. OK, you get the inequality

`y \ge 2x+3`.

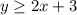The graph will be a half-plane -- all the points on one side of a line. The line that is the boundary of the half-plane has an equation:
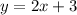`y=2x+3` -- just use an = sign instead of the inequality sign.

Graph the line.

The equation of the line is in slope-intercept form: y = mx + b, so you can tell the y-intercept is 3 and the slope is 2 (think of it as a fraction 2/1). Graph the line by going to the point (0, 3) -- the y-intercept -- then use the slope 2/1 interpreted as "rise over run" to go up 2 units and right 1 unit, arriving at the point (1, 5). Draw the line through those points, (0, 3) and (1, 5).

Now you have to decide which side of the line the inequality
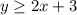`y \ge 2x+3` is describing. To do this, pick a point which is not on the line, plug its coordinates into the inequality; if the result is true, shade the side of the line the point you picked is on (if false, shade the other side!)

An easy point to pick in this case is the origin, (0, 0). Put zeros in for x and y in the inequality, and you'll get the statement
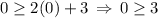`0 \ge 2(0)+3 \, \Rightarrow \, 0 \ge 3`. That's false, so shade the side of the line not containing the origin. In the image below, the shading is in purple.

All right, now for the other inequality,
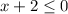`x+2\le 0`. Subtract 2 from both sides and the inequality becomes
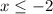`x \le -2`. This, too, graphs as a half-plane whose boundary line has equation
`x=-2`. Graph the line. A line with an equation that has x in it but not y is a vertical line with all its x-coordinates equal to the number on the right side of the equation. This line is vertical and goes through points such as (-2, 0).

Pick a point not on the line (the origin works again). Put the coordinates into the inequality to get
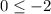`0\le -2` which is false. Shade the side of the vertical line which does not contain the origin. In the image below, the shading is in black.

Finally, YAY! \o/ , the solutions to the system are all the points in the plane that got shaded twice. In the image, they are the cross-hatched points above the purple line and to left of the black line.

Note: If you get a system with three inequalities, you'll be graphing three half-planes and looking for points that got shaded three times!

Note: One of your questions has the inequalities
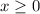`x \ge 0` and
`y \ge 0` in it. These two inequalities say that the x and y coordinates are both positive or zero, confining your attention to Quadrant I in the upper-right part of a graph, above the x-axis and to the right of the y-axis.