Answer:
A.

Explanation:
A. The given options are;

For
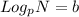 we have;
we have;

However;
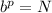
Where, p ≠ b
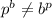
Therefore;
N ≠ N for the given pair and the pair are therefore, not equivalent
B. x = √y and
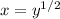
We note that
x = √y =
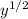
We have;
x =
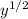 , by transitive property
, by transitive property
Therefore, the pair, x = √y and
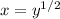 are equivalent
are equivalent
C.
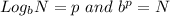
From
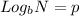 , we have;
, we have;
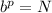
Therefore,
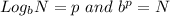 are equivalent pairs as
are equivalent pairs as
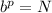 can be obtained from
can be obtained from
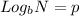
D. ㏑x = y and x =
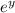
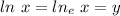 , therefore, by rules of logarithm, we have;
, therefore, by rules of logarithm, we have;
x =
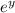
Therefore, ㏑x = y and x =
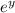 are equivalent pairs, as x =
are equivalent pairs, as x =
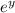 can be obtained from ln x.
can be obtained from ln x.
Therefore;
The option which are not equivalent pair is option is
