Question

Fast Auto Service provides a lubrication service for cars. It is known that the mean time taken for this

service is 15 minutes per car and the standard deviation is 2.4 minutes. Assume that the times taken for the service for all cars have a normal distribution If a service time is selected at random, find the
probability that the time is:
(i) more than 18 minutes
(ii) less than 10 minutes
(iii) between 12 and 16 minutes (10 marks)



please help me answer this question my people

Answer 1

The answer is below

Step-by-step explanation:

The z core is used to determine by how many standard deviations the raw score is above or below the mean. The z score is given by:

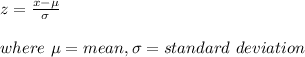`z=(x-\mu)/(\sigma)\\\\where\ \mu=mean,\sigma=standard\ deviation`, x = raw score

Given that mean (μ) = 15 minutes per car, standard deviation (σ) = 2.4 minutes.

1) For x > 18:

`z=(x-\mu)/(\sigma) =(18-15)/(2.4) =1.25`

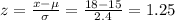
From normal distribution table, P(x > 18) = P(z > 1.25) = 1 - P(z < 1.25) = 1 - 0.8944 = 0.1056

2) For x < 10:

`z=(x-\mu)/(\sigma) =(10-15)/(2.4) =-2.08`

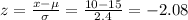
From normal distribution table, P(x < 10) = P(z < -2.08) = 0.0188

3) For x > 12:

`z=(x-\mu)/(\sigma) =(12-15)/(2.4) =-1.25`

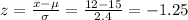
For x < 16:

`z=(x-\mu)/(\sigma) =(16-15)/(2.4) =0.42`

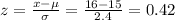
From normal distribution table, P(12 < x < 16) = P(z < 0.42) - P(z < -1.25) = 0.6628 - 0.1056 = 0.5572