Question

Please help i dont understand​

Answer 1

Given:

A quadrilateral inscribed in a circle.

To find:

The value of x and y.

Solution:

If a quadrilateral inscribed in a circle, then it is known as cyclic quadrilateral and the opposite angles of a cyclic quadrilateral are supplementary angles, it means their sum is 180 degrees.

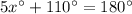`5x^\circ+110^\circ=180^\circ` [Supplementary angles]

`5x^\circ=180^\circ -110^\circ`

`x^\circ=(70^\circ)/(5)`

`x^\circ=14^\circ`

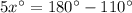
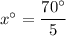
The value of x is 14 degrees.

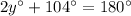`2y^\circ+104^\circ=180^\circ` [Supplementary angles]

`2y^\circ=180^\circ -104^\circ`

`y^\circ=(76^\circ)/(2)`

`y^\circ=38^\circ`

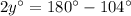
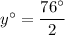
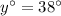
Therefore, the value of x is 14 degrees and the value of y is 38 degrees.