Answer:
See answers below
Explanation:
Problem 1
Recall that
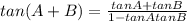 and that
and that
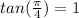 . Using these two facts, we can rewrite the expression:
. Using these two facts, we can rewrite the expression:

Hence, the first choice is correct
Problem 2
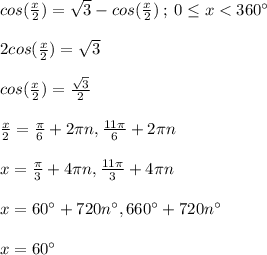
It's helpful to use the unit circle to solve these kinds of problems. Therefore, the third answer is correct.
Problem 3
Because
 and our parameters are
and our parameters are
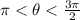 , the triangle must be in Quadrant III where
, the triangle must be in Quadrant III where
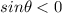 and
and
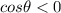 .
.
You may recall the double angle formula
 . We can find
. We can find
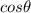 using
using
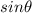 with the Pythagorean Identity
with the Pythagorean Identity
 keeping our parameters in mind:
keeping our parameters in mind:
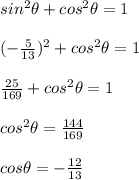
Thus,
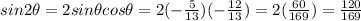 , which means the third option is correct.
, which means the third option is correct.