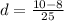Answer:
The probability you will randomly select a green and yellow treat is 2/25 greater than an orange and yellow treat
Step-by-step explanation:
Given
Let
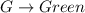
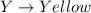
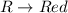
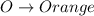

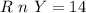
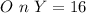
See comment for complete question
Required
How much greater is
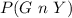 than
than
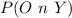
First, calculate
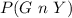 and
and
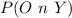
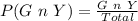
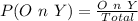
Where
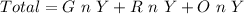

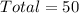
So:
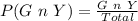
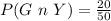

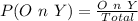

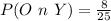
Calculate the difference (d) between both
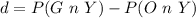

Take LCM