Question

Solve the equation for all real solutions.

Answer 1

`d=(5)/(2),\\\\d=(2)/(5)`

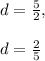
Explanation:

You can use the quadratic formula for any quadratic. The quadratic formula is given by
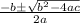`(-b\pm√(b^2-4ac))/(2a)`, and yields both real and non-real solutions.

In the given equation
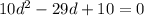`10d^2-29d+10=0`, we have:

`a=10,\\b=-29,\\c=10`

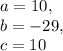
Substituting given values into the quadratic formula, we have:

`x=(29\pm√((-29)^2-4(10)(10)))/(2(10)),\\\\x=(29\pm√(441))/(20),\\\\x=(29\pm 21)/(20),\\\\\begin{cases}x=(29+21)/(20),x=(50)/(20)=\boxed{(5)/(2)}\\x=(29-21)/(20), x=(8)/(20)=\boxed{(2)/(5)}\end{cases}`

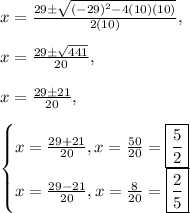
The attempt in the problem is correct. Sometimes with auto-grading, the solutions need to be in a specific order to be marked correct. I'd suggest submitting the solutions in a different order to check if that's the issue.