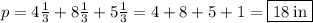Question

Please help on this...
Fine the perimeter and the area of this.

Answer 1

`p=18\:\mathrm{in},\\A=25\:\mathrm{in^2}`

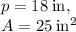
Explanation:

The area of a triangle is given by
`A=(1)/(2)\cdot b\cdot h`. By definition, the base and height must intersect at a
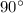`90^(\circ)` angle.

In this diagram, we notice that the lengths
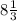`8(1)/(3)` and
`6` intersect at a
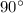`90^(\circ)`, so we can use them as the base and height of the triangle, respectively.

Therefore, the area of the triangle is equal to:

`A=(1)/(2)\cdot 6\cdot 8(1)/(3)=3\cdot (25)/(3)=\boxed{25\:\mathrm{in^2}}`.

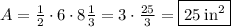The perimeter is simply the sum of all sides of the triangle. Thus, we have:

`p=4(1)/(3)+8(1)/(3)+5(1)/(3)=4+8+5+1=\boxed{18\:\mathrm{in}}`

Answer 2

p = 18 in

a = 25 in²

Explanation:

perimeter = sum of the sides

p = 4 1/3 + 5 1/3 + 8 1/3

p = 18 in

Area = 1/2 * base * height

a = 1/2 * 8 1/3 * 6

a = 1/2 * 50

a = 25 in²