Answer:
BC ≈ 4.0
Explanation:
∠ DCA = 180° - 70° = 110° ( adjacent angles )
∠ DAC = 180° - (30 + 110)° ← sum of angles in triangle
∠ DAC = 180° - 140° = 40°
Using the Sine rule in Δ ACD to find common side AC
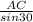 =
=
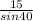 ( cross- multiply )
( cross- multiply )
AC × sin40° = 15 × sin30° ( divide both sides by sin40° )
AC =
 ≈ 11.668
≈ 11.668
Using the cosine ratio in right triangle ABC
cos70° =
 =
=
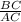 =
=
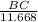 ( multiply both sides by 11.668 )
( multiply both sides by 11.668 )
11.668 × cos70° = BC , then
BC ≈ 4.0 ( to the nearest tenth )