Question

The 2nd term of an arithmetic progression is (1/2) and the sum of the first 14th terms is -70. Find

(a) the common difference
(b) the last term.​

Answer 1

The common difference is -1.

The last term (the 14th term) is -11.5.

Explanation:

In an arithmetic sequence, the second term is 0.5 and the sum of the first 14 terms is -70.

We want to determine the: (a) common difference and (b) the last term.

We can write an explicit formula to represent the sequence. An arithmetic sequence can be modeled by the formula:

`\displaystyle x_n=a+d(n-1)`

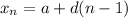
Where a is the initial term, d is the common difference, and n represents the nth term.

Since the second term is 0.5:

`x_2=0.5=a+d(2-1)`

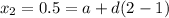
Simplify:

`x_2=0.5=a+d`

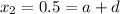
The sum of an arithmetic sequence is given by the formula:

`\displaystyle S=(k)/(2)\left(a+x_k\right)`

Where k is the number of terms and x_k is the last term.

Since the sum of the first 14 terms is -70, S = -70 and k = 14:

Using our explicit formula, the last term is:

`x_(14)=a+d(14-1)=a+13d`

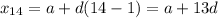
Substitute:

`\displaystyle -70=(14)/(2)(a+(a+13d))`

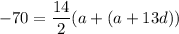
Simplifiy:

`-10=2a+13d`

Rewrite the equation for the second term:

`a=0.5-d`

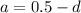
Substitute:

`-10=2(0.5-d)+13d`

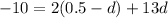
Simplify:

`-10=1-2d+13d`

Solve for d:

`d=-1`

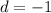
Hence, our common difference is -1.

Solve for a, the initial term:

`a=0.5-(-1)=1.5`

So, our explicit formula is now:

`x_n=1.5-1(n-1)=1.5-n+1=2.5-n`

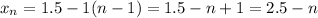
So, the last term (which is 14) is:

`x_(14)=2.5-(14)=-11.5`