Question

An object is traveling on a circle with a radius of 6 feet. If in 80 seconds a central angle of 9/4 radians is swept out, then find both the angular speed and the linear speed of the object. Give exact answers. DO NOT provide a decimal approximation.

Answer 1

The angular speed of the object is 0.0281 rad/s

The linear speed of the object is 0.169 ft/s

Step-by-step explanation:

Given;

radius of the circle, r = 6 ft

time of motion of the object around the circle, t = 80 s

central angle formed by the object during the motion, θ = 9/4 rad = 2.25 rad

The angular speed of the object is calculated as;

`\omega = (\theta )/(t) = (2.25 \ rad)/(80 \ s) = 0.0281 \ rad/s`

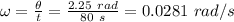
The linear speed of the object is calculated as;

v = ωr

v = 0.0281 rad/s x 6ft

v = 0.169 ft/s